8.1.1.2.2 - Example with Summarized Data.8.1.1.2.1 - Example with Summarized Data.8.1.1.2 - Minitab: Confidence Interval for a Proportion.8.1.1.1.2 - Video Example: Dog Ownership.8.1.1.1.1 - Video Example: PA Residency.8.1.1.1 - Normal Approximation Formulas.7.4.2.3 - Example: 99% CI for Proportion of Women Students.7.4.2.2 - Video Example: 90% CI for the Correlation between Height and Weight.7.4.2.1 - Video Example: 98% CI for Mean Atlanta Commute Time.7.4.1.6 - Example: Difference in Mean Commute Times.7.4.1.4 - Example: Proportion of Women Students.7.4.1.3 - Example: Proportion NFL Coin Toss Wins.7.4.1.2 - Video Example: Correlation Between Printer Price and PPM.7.4.1.1 - Video Example: Mean Body Temperature.7.3 - Minitab: Finding Values Given Proportions.7.2.3.1 - Example: Proportion Between z -2 and +2.7.2 - Minitab: Finding Proportions Under a Normal Distribution.6.6 - Confidence Intervals & Hypothesis Testing.5.5.4 - Correlation Example: Quiz & Exam Scores.5.5.3 - Difference in Means Example: Exercise by Biological Sex.5.5.1 - Single Proportion Example: PA Residency.5.5 - Randomization Test Examples in StatKey.
5.3.1 - StatKey Randomization Methods (Optional). 5.1 - Introduction to Hypothesis Testing. 4.6 - Impact of Sample Size on Confidence Intervals. 4.4.2.2 - Example: Difference in Dieting by Biological Sex. 4.4.2.1 - Example: Correlation Between Quiz & Exam Scores. 4.4.1.2 - Example: Difference in Mean Commute Times. 4.4.1.1 - Example: Proportion of Lactose Intolerant German Adults. 4.3.2 - Example: Bootstrap Distribution for Difference in Mean Exercise. 4.3.1 - Example: Bootstrap Distribution for Proportion of Peanuts. 4.2.1 - Interpreting Confidence Intervals. 4.2 - Introduction to Confidence Intervals. 4.1.1.2 - Coin Flipping (One Proportion). 3.5 - Relations between Multiple Variables. 3.4.2.2 - Example of Computing r by Hand (Optional). 3.4.2.1 - Formulas for Computing Pearson's r. 3.3 - One Quantitative and One Categorical Variable. 2.2.6 - Minitab: Central Tendency & Variability. 2.2.1 - Graphs: Dotplots and Histograms. 2.1.3.2.5.1 - Advanced Conditional Probability Applications. 2.1.3.2.1 - Disjoint & Independent Events. 
2.1.2.1 - Minitab: Two-Way Contingency Table. 1.2.2.1 - Minitab: Simple Random Sampling. 1.1.2 - Explanatory & Response Variables. 1.1.1 - Categorical & Quantitative Variables. This is also known as an indirect relationship.Ī bivariate outlier is an observation that does not fit with the general pattern of the other observations. For example, as values of x get larger values of y get smaller. The linear relationship between two variables is negative when one increases as the other decreases. This is also known as a direct relationship. The linear relationship between two variables is positive when both increase together in other words, as values of x get larger values of y get larger. This occurs when the line-of-best-fit for describing the relationship between x and y is a straight line.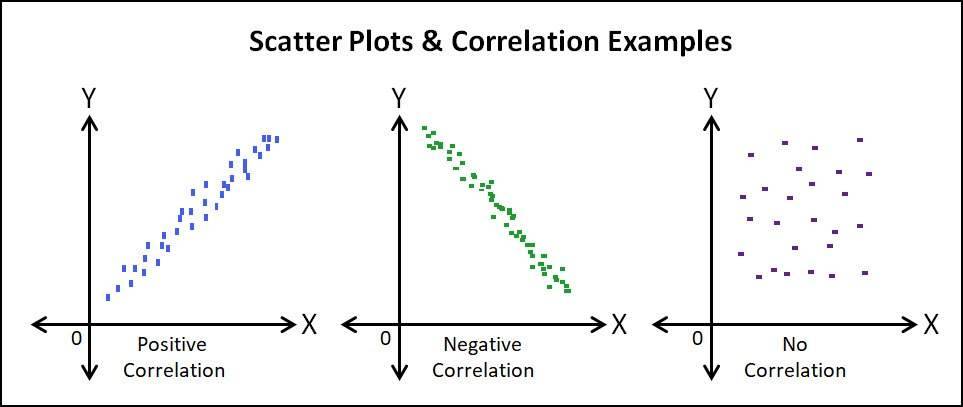 
In this class, we will focus on linear relationships. When examining a scatterplot, we need to consider the following: Scatterplot A graphical representation of two quantitative variables in which the explanatory variable is on the x-axis and the response variable is on the y-axis.
0 kommentar(er)
